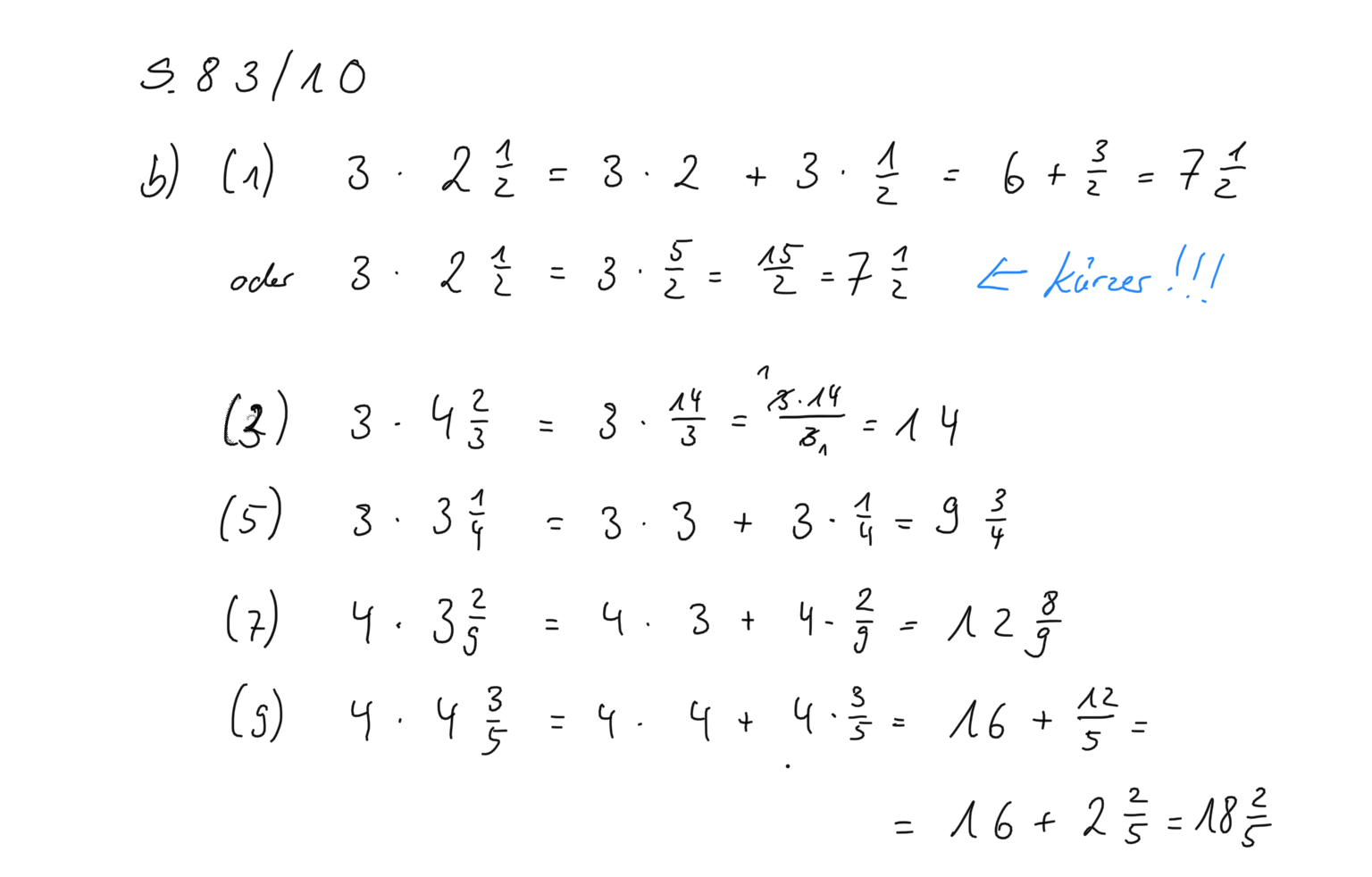M6 3.1 Vervielfachen und Teilen von Brüchenl

S.82/6
S.82/3b,c
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle 4 \cdot {2 \over 5}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mn>4</mn> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>5</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle 4 \cdot {2 \over 5}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=71bcf03fe138f8dd763bb33828a0e56d&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 4.84ex;" alt="{\displaystyle 4 \cdot {2 \over 5}}"></span>
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle 16 \cdot {5 \over 56}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mn>16</mn> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>5</mn> <mn>56</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle 16 \cdot {5 \over 56}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=2046b289c5b8ab52520e1a48f4cc0491&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 7.165ex;" alt="{\displaystyle 16 \cdot {5 \over 56}}"></span>
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle 3 \cdot {5 \over 6}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mn>3</mn> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>5</mn> <mn>6</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle 3 \cdot {5 \over 6}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=2085c228ede958ec10cd08dce94aec0b&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 4.84ex;" alt="{\displaystyle 3 \cdot {5 \over 6}}"></span>
Berechne folgende Aufgaben im Kopf und kürze, falls möglich.<br> <span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle 3 \cdot {1 \over 4}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mn>3</mn> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mn>4</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle 3 \cdot {1 \over 4}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=f62e7531bc81ec9cab73c893dd46fc96&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 4.84ex;" alt="{\displaystyle 3 \cdot {1 \over 4}}"></span>
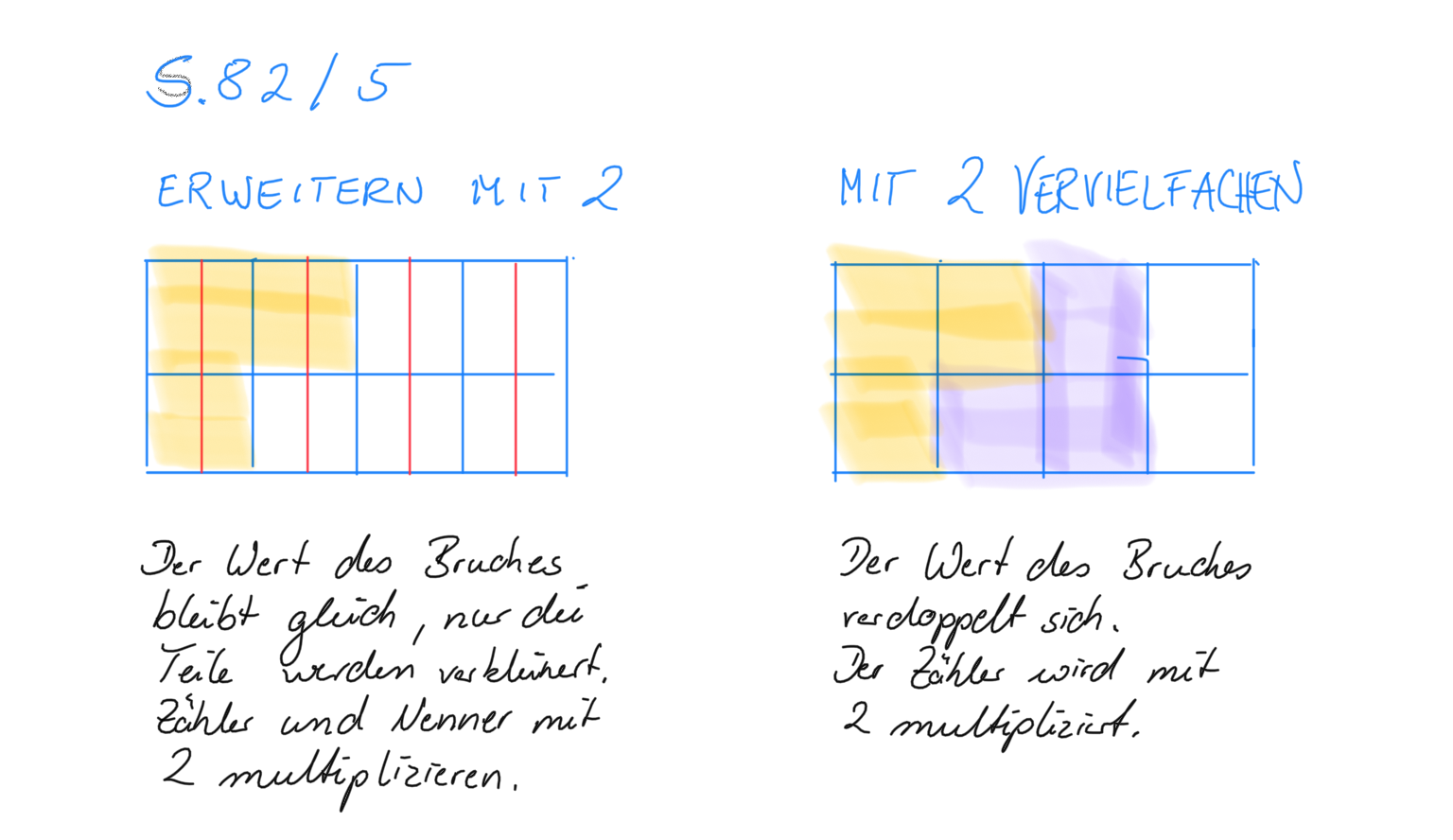
Bearbeite dazu S.82/5
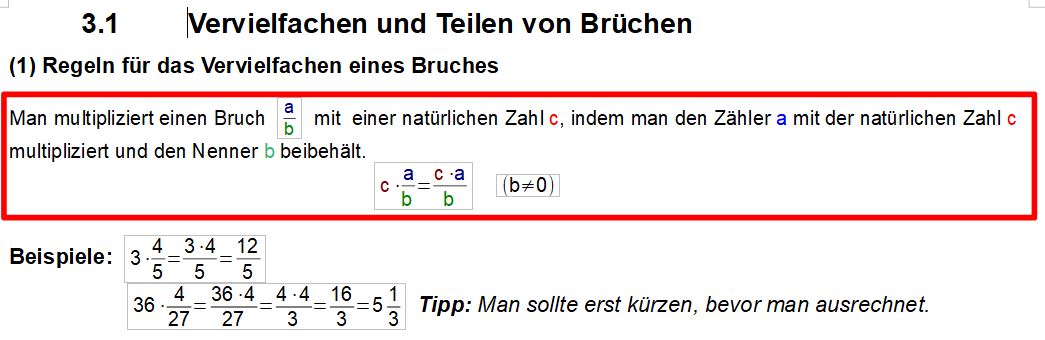
Wir brauchen uns nur den Zähler anzuschauen, wenn der Nenner gleich ist. Denn beim Vervielfachen ändert man den Nenner nicht und nur der Zähler wird vervielfacht.
Brüche in unechte Brüche umwandeln, wenn nötig.
Brüche auf den gemeinsamen Nenner bringen, wenn nötig.
Nun schauen wir uns nur den Zähler an, wenn ihr nicht jetzt schon seht, welchen Wert für x in Frage kommt.
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle x \cdot {1 \over 4}= 1 {3 \over 4}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>x</mi> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mn>4</mn> </mfrac> </mrow> <mo>=</mo> <mn>1</mn> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>3</mn> <mn>4</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle x \cdot {1 \over 4}= 1 {3 \over 4}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=052e342552a6232f73f3c2842290ed58&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 11.267ex;" alt="{\displaystyle x \cdot {1 \over 4}= 1 {3 \over 4}}"></span>
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle x \cdot {2 \over 5}= {2 \over 10}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>x</mi> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>5</mn> </mfrac> </mrow> <mo>=</mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>10</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle x \cdot {2 \over 5}= {2 \over 10}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=f73508b942e3a8c70931193595bb7dc3&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 11.267ex;" alt="{\displaystyle x \cdot {2 \over 5}= {2 \over 10}}"></span>
Berechne folgende Aufgaben im Kopf und kürze, falls möglich.<br> <span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle x \cdot {1 \over 4}= {2 \over 4}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>x</mi> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mn>4</mn> </mfrac> </mrow> <mo>=</mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>4</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle x \cdot {1 \over 4}= {2 \over 4}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=5cabadc4450531efc0258803845eb265&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 10.104ex;" alt="{\displaystyle x \cdot {1 \over 4}= {2 \over 4}}"></span>
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle x \cdot {2 \over 8}= {2 \over 4}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>x</mi> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>8</mn> </mfrac> </mrow> <mo>=</mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>4</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle x \cdot {2 \over 8}= {2 \over 4}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=631004ed49fceedcf8f7749d0996ae81&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 10.104ex;" alt="{\displaystyle x \cdot {2 \over 8}= {2 \over 4}}"></span>
S.82/9
Inhaltsverzeichnis
Teil 2 Donnerstag 17.12.
Zur Vertiefung:
S.83/10a
Bei Marias Lösung ist wird die gemischte Schreibweise in die unechte Schreibweise umgewandelt und dann gerechnet.
Patrick verwendet das Distributivgesetz. Denn
Wende nun beide Rechenwege mindestens einmal an: S.83/10b Nummer (1),(3),(5),(7),(9)
S.82/8
Leo und seine 6 Freunde sind zusammen 7 Personen.
Bearbeite die folgenden Aufgaben in deinem Übungsheft und kontrolliere mit grün oder einem Glitzerstift.
Weihnachten steht endlich vor der Tür.
- In der Familie wird der Weihnachtspunsch zubereitet. Alle vier Personen trinken 3 achtel Liter Punsch. Berechne, wie viele Liter die Mutter kaufen muss.
- Nun haben sich die Eltern vom Vater angemeldet und wollen ebenfalls mittrinken. Beide trinken einen achtel Liter. Berechne, wie viele Liter der Vater noch dazu kaufen muss.
- Bei den Nachbarn trinken die 5 Familienmitglieder jeweils einen viertel Liter. Die Großeltern möchten drei sechzehntel Liter trinken. Berechne, wie viel Liter Punsch die Nachbarn kaufen müssen. Notiere einen ganzen Term.
- Der Punsch wird in Literflaschen zu 3,95 € verkauft. Berechne, wie viel die Nachbarn bezahlen müssen.
Die Nachbarn müssen 2 Falschen zu je 3,95€ kaufen.
Da wir noch keine Multiplikation von Dezimalbrüchen hatten, könnt ihr die Zahlen entweder addieren oder in Cent umrechnen.
- Addition: 3,95€ + 3,95€ = 7,90€
- in Cent:
cent = 790 cent = 7,90€
Zeichne in ein Koordinatensystem folgende Punkte:
- Das ganze soll ein Tannebaum werden. Gib die Koordinaten von E, F, M, N, P und S an und vervollständige das Bild.
- An der Stelle
hängt eine rote Kugel. Zeichne sie ein.
- Du kannst den Baum nun verzieren und anmalen.
- Schicke mir ein Foto von deinem Tannebaum. Ich freue mich schon.
Wichtig! Diese Aufgabe ist freiwillig. S.83/13
Wenn ihr eine andere Lösung habt, dann könnt ihr mir diese gerne schicken.
a) Der Nenner kann ein ganz anderer sein.
Also der Nenner ist immernoch 2 und 15 ist um 10 größer als 5.
b)
Teil 3: Freitag 18.12. wiederholen
Potenzen werden genutzt, um Aufgaben kürzer darzustellen. Anstatt eines mit mehreren gleichen kann man auch Potenzen schreiben. Beim Potenzieren gibt den Faktor an und gibt dieser an.
Faktorendie BasisProduktesder Exponent
Berechne folgende Potenzen. Schreibe dazu zuerst als Produkt.
Beispiel:
a) b)
c)
d)
e)
a)
b)
c)
d)
Mittwoch, 11.01.2021
Mal schauen, ob du es verstanden hast.
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {2 \over 5} : 3 = {2 \over {5 \cdot 3}} }"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>5</mn> </mfrac> </mrow> <mo>:</mo> <mn>3</mn> <mo>=</mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mrow class="MJX-TeXAtom-ORD"> <mn>5</mn> <mo>⋅<!-- ⋅ --></mo> <mn>3</mn> </mrow> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {2 \over 5} : 3 = {2 \over {5 \cdot 3}} }</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=1ae4073f1a4a2d8375594ea63024adad&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 13.037ex;" alt="{\displaystyle {2 \over 5} : 3 = {2 \over {5 \cdot 3}} }"></span> =
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {3 \over 7} : 4 }"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>3</mn> <mn>7</mn> </mfrac> </mrow> <mo>:</mo> <mn>4</mn> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {3 \over 7} : 4 }</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=d1800c8484f58944211374c8505b28c8&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -2.005ex; height: 5.343ex; width: 5.098ex;" alt="{\displaystyle {3 \over 7} : 4 }"></span> =
Teilen von Brüchen - Wähle die richtige Lösung aus.<br> <span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {1 \over 3} : 2 = {1 \over {3 \cdot 2}} }"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mn>3</mn> </mfrac> </mrow> <mo>:</mo> <mn>2</mn> <mo>=</mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mrow class="MJX-TeXAtom-ORD"> <mn>3</mn> <mo>⋅<!-- ⋅ --></mo> <mn>2</mn> </mrow> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {1 \over 3} : 2 = {1 \over {3 \cdot 2}} }</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=484d98d2d6cb56f298b9a0af2948824a&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 13.037ex;" alt="{\displaystyle {1 \over 3} : 2 = {1 \over {3 \cdot 2}} }"></span> =
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {12 \over 5} : 4 }"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>12</mn> <mn>5</mn> </mfrac> </mrow> <mo>:</mo> <mn>4</mn> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {12 \over 5} : 4 }</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=ad3cda1c10dd6a866e528e49613454be&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 6.261ex;" alt="{\displaystyle {12 \over 5} : 4 }"></span>=
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {4 \over 5} : 12 }"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>4</mn> <mn>5</mn> </mfrac> </mrow> <mo>:</mo> <mn>12</mn> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {4 \over 5} : 12 }</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=9ee9fcc5f3d5f60fa89d32ad0c02d0cf&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 6.261ex;" alt="{\displaystyle {4 \over 5} : 12 }"></span>=
Denke nun auch an das Kürzen vor dem Ausrechnen. <br> <span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {2 \over 3} : 2 }"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>3</mn> </mfrac> </mrow> <mo>:</mo> <mn>2</mn> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {2 \over 3} : 2 }</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=9be88bf49882cef5d3dbe563263a3054&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 5.098ex;" alt="{\displaystyle {2 \over 3} : 2 }"></span> =
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {6 \over 5} : 3 }"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>6</mn> <mn>5</mn> </mfrac> </mrow> <mo>:</mo> <mn>3</mn> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {6 \over 5} : 3 }</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=174d3263e86fcd389a911cacf34dd1f6&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 5.098ex;" alt="{\displaystyle {6 \over 5} : 3 }"></span>=
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {5 \over 7} : 5 }"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>5</mn> <mn>7</mn> </mfrac> </mrow> <mo>:</mo> <mn>5</mn> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {5 \over 7} : 5 }</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=a6760fa729beda292271d2fab4a92bba&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -2.005ex; height: 5.343ex; width: 5.098ex;" alt="{\displaystyle {5 \over 7} : 5 }"></span>=
2 im Zähler und Nenner kann man kürzen.
Die 6 im Zähler wird nur 3 geteilt. (Hier kannst du auch im Buch S. 84 im roten Kasten nachlesen.
Nehmt nun euer Buch zur Hand und bearbeitet die folgenden Aufgaben.
S.85/ 6 (Notiert die Lösung im Übungsheft und vergleicht sie mit der Lösung hier.
a) ; b)
; c)
; d)
; e)
:
f)
; g)
; h)
; i)
; j)
;
Donnerstag 14.1. 2021
Wir treffen uns alle um 9:45 Uhr in eurem virtuellen Klassenzimmer. Für diejenigen, die sich noch unsicher sind, wiederhole ich noch einmal die Theorie von gestern. Danach können alle selbständig üben. Korrigiere nach jeder Aufgabe deine Lösung mit der Musterlösung.
- S. 84/3 (wie im Kasten rechts)
- S. 84/4
- S. 85/7 (Unterschied zwischen teilen und kürzen)
- S. 85/8b (5-8) und freiwillig kann man hier auch ganze Zahlen geschickt zerlegen (13)
- S. 85/9 a-d
- S. 85/11 (6 beliebige Aufgaben), 13
- Wichtige Aufgabe:
a) Berechne.
b) Notiere in dein Übungsheft, was diese Aufgabe mit der Aufgabezu tun haben könnte.
- Für schnelle freiwillig zum Knobeln. Tipp: Eine Tabelle für die drei Piraten hilft bei der Lösung. S.85/14 *
Lösungen findet ihr im Modul Lernen. Wie immer sorgfältig korrigieren. Die wichtige Aufgabe besprechen wir morgen gemeinsam.
Zur Abwechselung:
Freitag, 15.1.2021
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {2 \over 3} \cdot {2 \over 3}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>3</mn> </mfrac> </mrow> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>3</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {2 \over 3} \cdot {2 \over 3}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=3cf32367b0765c1ad5e4e28a0c6997f2&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 5.676ex;" alt="{\displaystyle {2 \over 3} \cdot {2 \over 3}}"></span>=
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {1 \over 8} \cdot {5 \over 3}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mn>8</mn> </mfrac> </mrow> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>5</mn> <mn>3</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {1 \over 8} \cdot {5 \over 3}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=9ad9be62f21a9864527ccbb5f3a6fc40&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 5.676ex;" alt="{\displaystyle {1 \over 8} \cdot {5 \over 3}}"></span>=
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {15 \over 14} \cdot {9 \over 8}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>15</mn> <mn>14</mn> </mfrac> </mrow> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>9</mn> <mn>8</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {15 \over 14} \cdot {9 \over 8}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=9d16194dec3eff41b756cb113097739b&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 6.839ex;" alt="{\displaystyle {15 \over 14} \cdot {9 \over 8}}"></span> =
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle {3 \over 7} \cdot {3 \over 5}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>3</mn> <mn>7</mn> </mfrac> </mrow> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>3</mn> <mn>5</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle {3 \over 7} \cdot {3 \over 5}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=704a9032b7c2f4d4ad6e00581dbb9a45&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -2.005ex; height: 5.343ex; width: 5.676ex;" alt="{\displaystyle {3 \over 7} \cdot {3 \over 5}}"></span>=
Bearbeite selbstständig folgende Aufgaben:
- S.90/5
- S.91/10 (Suche dir 10 Aufgaben aus!)