6B Vor Weihnachten: Unterschied zwischen den Versionen
Keine Bearbeitungszusammenfassung Markierung: 2017-Quelltext-Bearbeitung |
Keine Bearbeitungszusammenfassung Markierung: 2017-Quelltext-Bearbeitung |
||
| Zeile 37: | Zeile 37: | ||
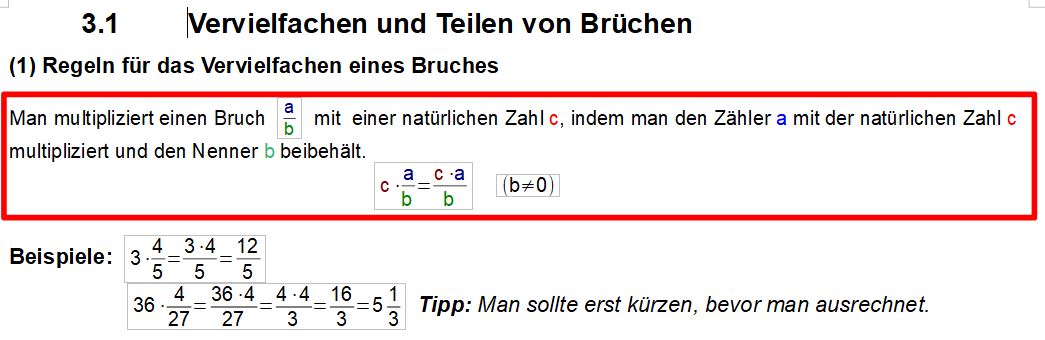
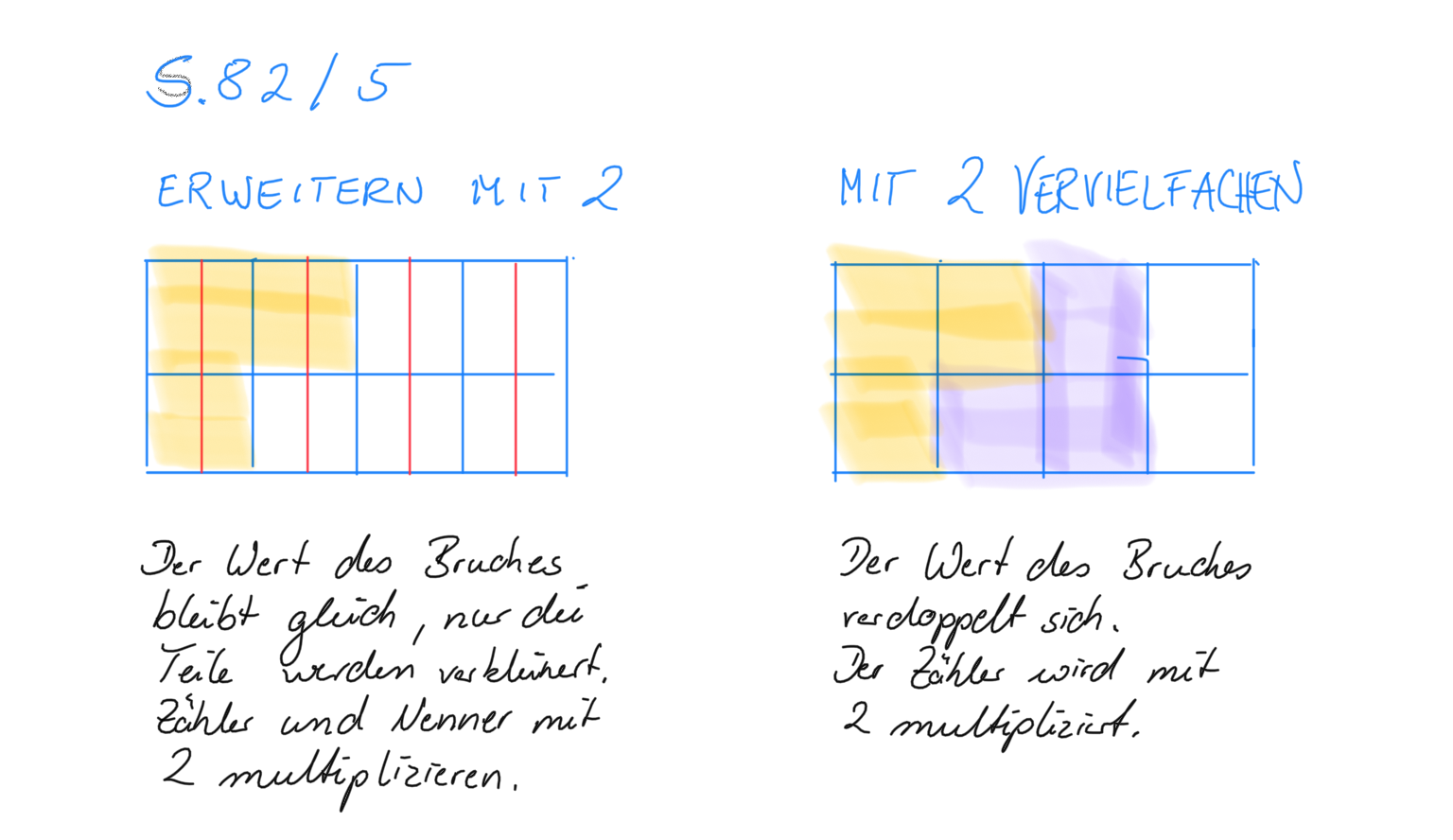
{{Box|1=Vergleiche das Erweitern mit dem Vervielfachen!|2= | {{Box|1=Vergleiche das Erweitern mit dem Vervielfachen!|2= | ||
Bearbeite | Bearbeite im Übungsheft und vergleiche dann deine Lösung. <br> | ||
S.82/5 | |||
{{Lösung versteckt|1=[[Datei:PNG-Bild 2.png|midi]]|2=Aufdecken|3=Verbergen}} | {{Lösung versteckt|1=[[Datei:PNG-Bild 2.png|midi]]|2=Aufdecken|3=Verbergen}} | ||
|3=Üben}} | |3=Üben}} | ||
| Zeile 59: | Zeile 60: | ||
<math> x \cdot {2 \over 5}= {2 \over 10}</math> (!x = 1) (x= 2) (!x=4) | <math> x \cdot {2 \over 5}= {2 \over 10}</math> (!x = 1) (x= 2) (!x=4) | ||
<math> x \cdot {1 \over 4}= 1 {3 \over 4}</math> (!x = | <math> x \cdot {1 \over 4}= 1 {3 \over 4}</math> (!x = 2) (x= 7) (!x=3) | ||
</div> | </div> | ||
|3=Unterrichtsidee}} | |3=Unterrichtsidee}} | ||
{{Box|1=Jetzt bist du nochmal dran!|2= | {{Box|1=Jetzt bist du nochmal dran!|2= | ||
Bearbeite im Übungsheft und vergleiche dann deine Lösung. | |||
S.82/9 | S.82/9 | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
Version vom 15. Dezember 2020, 12:38 Uhr
Einen schönen guten Morgen!
S.82/6
S.82/3b,c
Berechne folgende Aufgaben im Kopf und kürze, falls möglich.<br> <span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle 3 \cdot {1 \over 4}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mn>3</mn> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mn>4</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle 3 \cdot {1 \over 4}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=f62e7531bc81ec9cab73c893dd46fc96&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 4.84ex;" alt="{\displaystyle 3 \cdot {1 \over 4}}"></span>=
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle 3 \cdot {5 \over 6}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mn>3</mn> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>5</mn> <mn>6</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle 3 \cdot {5 \over 6}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=2085c228ede958ec10cd08dce94aec0b&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 4.84ex;" alt="{\displaystyle 3 \cdot {5 \over 6}}"></span> =
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle 4 \cdot {2 \over 5}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mn>4</mn> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>5</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle 4 \cdot {2 \over 5}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=71bcf03fe138f8dd763bb33828a0e56d&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 4.84ex;" alt="{\displaystyle 4 \cdot {2 \over 5}}"></span>=
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle 16 \cdot {5 \over 56}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mn>16</mn> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>5</mn> <mn>56</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle 16 \cdot {5 \over 56}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=2046b289c5b8ab52520e1a48f4cc0491&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 7.165ex;" alt="{\displaystyle 16 \cdot {5 \over 56}}"></span>=
Bearbeite im Übungsheft und vergleiche dann deine Lösung.
S.82/5
Wir brauchen uns nur den Zähler anzuschauen, wenn der Nenner gleich ist. Denn beim Vervielfachen ändert man den Nenner nicht und nur der Zähler wird vervielfacht.
Brüche in unechte Brüche umwandeln, wenn nötig.
Brüche auf den gemeinsamen Nenner bringen, wenn nötig.
Nun schauen wir uns nur den Zähler an, wenn ihr nicht jetzt schon seht, welchen Wert für x in Frage kommt.
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle x \cdot {2 \over 5}= {2 \over 10}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>x</mi> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>5</mn> </mfrac> </mrow> <mo>=</mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>10</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle x \cdot {2 \over 5}= {2 \over 10}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=f73508b942e3a8c70931193595bb7dc3&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 11.267ex;" alt="{\displaystyle x \cdot {2 \over 5}= {2 \over 10}}"></span>
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle x \cdot {1 \over 4}= 1 {3 \over 4}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>x</mi> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mn>4</mn> </mfrac> </mrow> <mo>=</mo> <mn>1</mn> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>3</mn> <mn>4</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle x \cdot {1 \over 4}= 1 {3 \over 4}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=052e342552a6232f73f3c2842290ed58&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 11.267ex;" alt="{\displaystyle x \cdot {1 \over 4}= 1 {3 \over 4}}"></span>
<span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle x \cdot {2 \over 8}= {2 \over 4}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>x</mi> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>8</mn> </mfrac> </mrow> <mo>=</mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>4</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle x \cdot {2 \over 8}= {2 \over 4}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=631004ed49fceedcf8f7749d0996ae81&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 10.104ex;" alt="{\displaystyle x \cdot {2 \over 8}= {2 \over 4}}"></span>
Berechne folgende Aufgaben im Kopf und kürze, falls möglich.<br> <span class="mwe-math-element"><span class="mwe-math-mathml-inline mwe-math-mathml-a11y" style="display: none;"><math xmlns="http://www.w3.org/1998/Math/MathML" alttext="{\displaystyle x \cdot {1 \over 4}= {2 \over 4}}"> <semantics> <mrow class="MJX-TeXAtom-ORD"> <mstyle displaystyle="true" scriptlevel="0"> <mi>x</mi> <mo>⋅<!-- ⋅ --></mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>1</mn> <mn>4</mn> </mfrac> </mrow> <mo>=</mo> <mrow class="MJX-TeXAtom-ORD"> <mfrac> <mn>2</mn> <mn>4</mn> </mfrac> </mrow> </mstyle> </mrow> <annotation encoding="application/x-tex">{\displaystyle x \cdot {1 \over 4}= {2 \over 4}}</annotation> </semantics> </math></span><img src="/index.php?title=Spezial:MathShowImage&hash=5cabadc4450531efc0258803845eb265&mode=mathml" class="mwe-math-fallback-image-inline" aria-hidden="true" style="vertical-align: -1.838ex; height: 5.176ex; width: 10.104ex;" alt="{\displaystyle x \cdot {1 \over 4}= {2 \over 4}}"></span>
Bearbeite im Übungsheft und vergleiche dann deine Lösung. S.82/9